Home

Episode 4 Supports

  • Episode Description

    Repeating Your Reasoning: Keoni and Sasha determine the equation of a parabola which has a vertex at (0,0) and distance of three units between its focus and vertex. Then they compare the equation to their conjectures from Episode 3.

     

  • Focus Questions

    For use in a classroom, pause the video and ask this question:

     

    1. [Pause video at 1:58]. For the quantity y – 3, where on the graph is the distance y? 3? y – 3?

     

  • Supporting Dialogue

    Provide the opportunity for involvement of all the students in the room by asking open-ended questions that can support many good observations:

     

    1. After Sasha and Keoni have developed two forms for the equation [4:01], ask “How do you know that each equation works?” If there is not an immediate response, ask students to share ideas with a neighbor.

    2. How do you know that the equations  x = √(12y) and y = x2/12 are equivalent?

  • Math Extensions

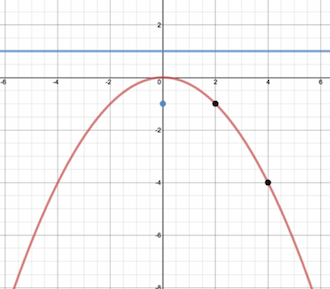
    Consider the circle below.

     

    1. Below is a graph of a parabola with a vertex at (0,0) and a focus at (0, –1). Using the definition of a parabola, find the coordinates of the two indicated points on the parabola.

     

    2. Explain how the coordinates of the points relate to the distances between the each point and the directrix and between the each point and the focus.

     

    ​

  •  

1

2

3

4

5

6

7

 

Mathematics in this Lesson

Lesson Description

Targeted Understanding

CC Math Standards

CC Math Practices

Lesson Description

 

Keoni and Sasha determine a general method for representing the equation for a parabola with a vertex on the origin and a focus p units above the vertex.

Targeted Understandings

 

This lesson can help students:

 

  • Interpret the meaning and use of an equation that they derive to represent a family of parabolas with vertex at the origin and focus p units above the vertex (y = x2/(4p)).

  • Conceive of the parameter p in the equation y =  x2/(4p)  as a distance between the vertex and focus of a parabola. The value of p can vary, but once it is set (e.g., when p = 2), then an equation is defined (e.g., y =  x2/8) that represents a unique parabola.

  • Relate geometric features of parabolas to elements of corresponding equations. For example, the equation y =  x2/8 can also be expressed as y =  x2/(2•4), where the 2 represents the number of units between the focus and vertex of the parabola.

Common Core Math Standards

• CCSS.M.HSG.GPE.A.2: Derive the equation of a parabola given a focus and directrix.

In Episode 6, Sasha and Keoni derive the equation (in two forms) for the family of parabolas with vertex (0,0) and focus p units above the vertex, namely y = x2/(4p)  and x = √(4py).  They do this by generalizing the method they used to develop an equation for a parabola with p = 1 in Lessons 3 and 4 (y =  x2/4 and x = √(4y)), for a parabola with p = 2 in Episode 2 of this lesson (y =  x2/8 and x = √(8y)), and for a parabola with p = 3 in Episode 4 (y =  x2/12 and x = √(12y)).

 

• CCSS.M.HSA.APR.A.1: Perform arithmetic operations on polynomials.

Sasha and Keoni multiply binomials, such as (y + 2)2 and (y + p)2, in service of deriving an equation for a particular parabola (in Episodes 2 and 4) or a family of parabolas (Episode 6).

 

 

 

Common Core Math Practices

 

CCSS.Math.Practice.MP7. Look for and make use of structure.

In this lesson, Sasha and Keoni make use of mathematical structure on two levels. First, they discern a pattern relating the equations of three parabolas (y = x2/4, y = x2/8, and y =  x2/12) with the corresponding distance between the vertex and focus (respectively, p = 1; p = 2; and p = 3).  Specifically, they see the structure of 1•4, 2•4 and 3•4 in the denominators of their equations, which is the p-value of the parabola multiplied by 4. Consequently Keoni and Sasha conjecture that the general equation of a parabola with vertex (0,0) and focus p units above the vertex will be y = x2/(4p).  Then they look for and make use of structure on a second level. To derive the equation y =  x2/(4p), they need to identify the lengths of the sides of a right triangle with hypotenuse connecting a general point on the parabola with the focus. To accomplish this challenging task, Sasha and Keoni see a pattern in similar quantities that they identified for particular parabolas, which they generalize to define lengths involving the parameter p, such as  y – p and y + p.